
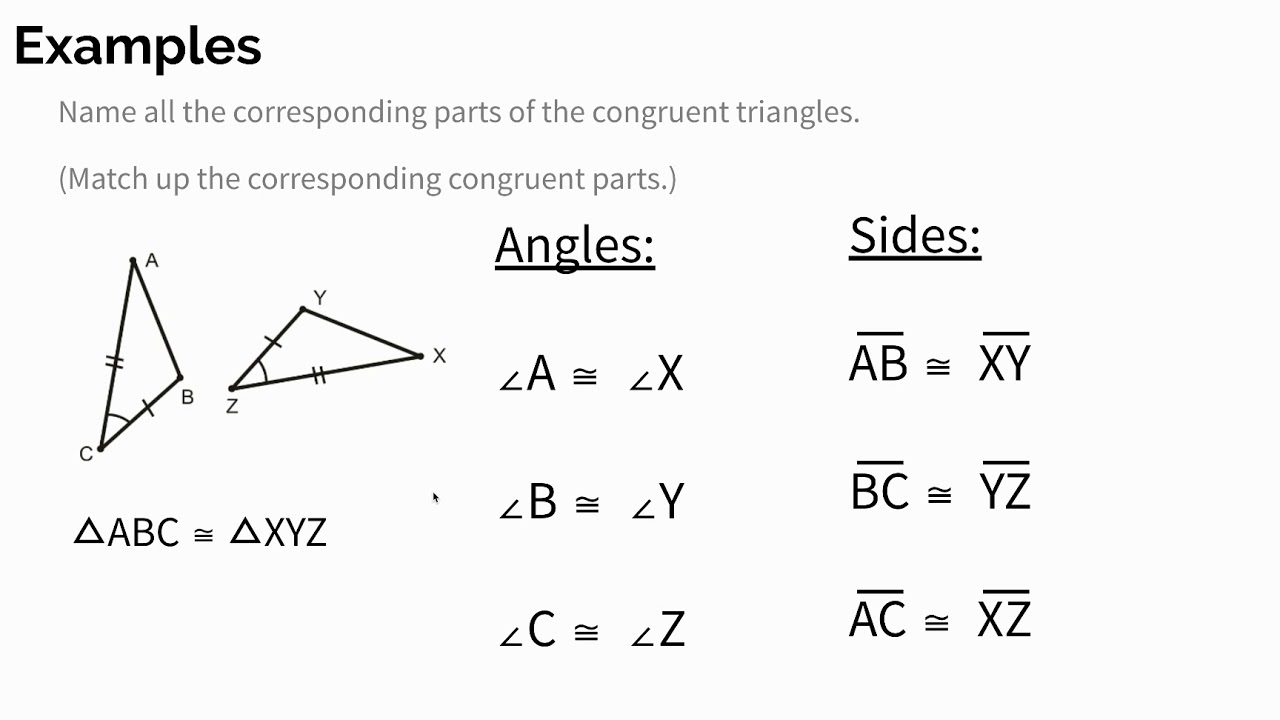
When two triangles are congruent, CPCTC ensures their corresponding sides are congruent. Let’s explore the properties of CPCTC in detail: Corresponding Sides The property of Corresponding Parts of Congruent Triangles are Congruent (CPCTC) states that if two triangles are congruent, their corresponding parts (sides, angles, and other elements) are also congruent. Once the congruence of the triangles is established, the proof applies the congruence relationships to the corresponding parts of the triangles, including sides, angles, and other elements. The proof of CPCTC relies on the foundation of triangle congruence, which can be established through different congruence criteria. Hence, we have successfully proven CPCTC, which states that corresponding parts of congruent triangles are congruent.Other corresponding elements, such as medians, altitudes, and diagonals, will also be congruent between the congruent triangles.Therefore, we have established that the corresponding sides and angles of congruent triangles ABC and DEF are congruent.
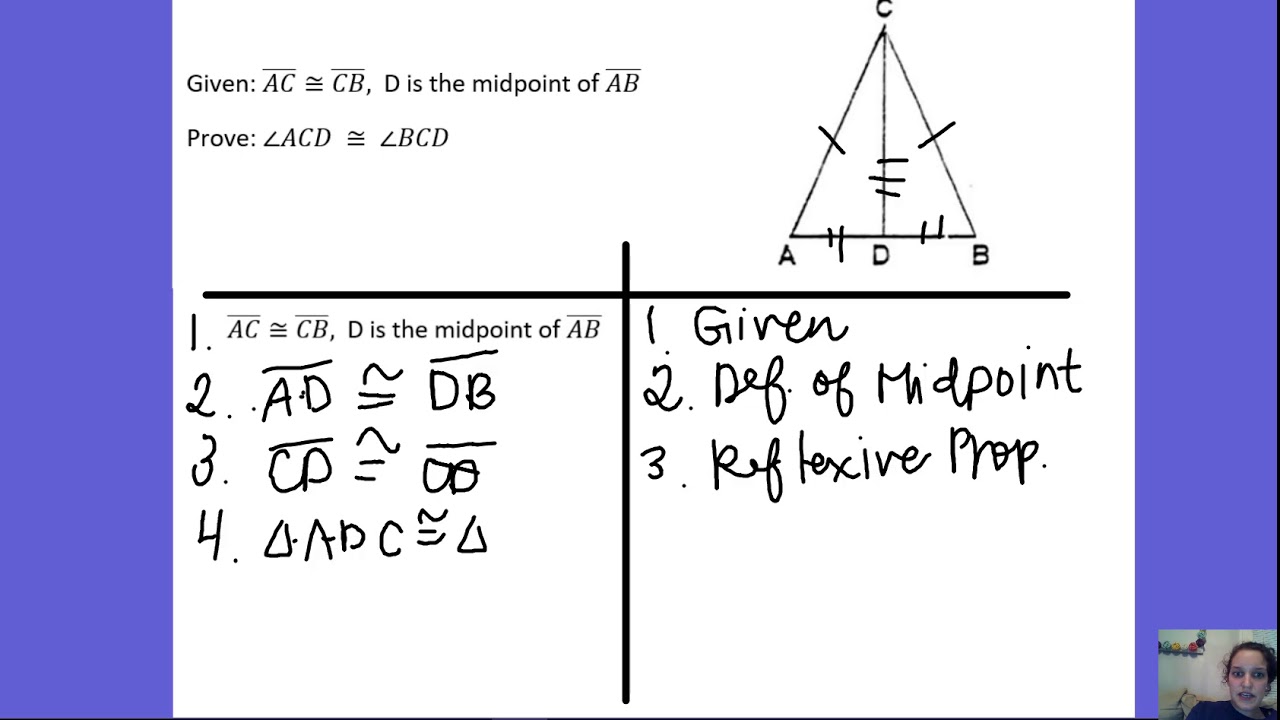
By the congruence of triangles ABC and DEF, we know that the corresponding angles are congruent:.Now, let’s consider the corresponding angles of the congruent triangles:.By the congruence of triangles ABC and DEF, we know that the corresponding sides are congruent:.Let’s consider the corresponding sides of the congruent triangles:.CPCTC states that congruent triangles’ corresponding sides, angles, and other elements are congruent.We want to prove: Corresponding parts of congruent triangles are congruent.Given that triangle, ABC is congruent to triangle DEF (proven using a congruence criterion such as SAS, SSS, or ASA).Let’s explore the proof of CPCTC in detail: The proof of CPCTC ( Corresponding Parts of Congruent Triangles are Congruent ) relies on the establishment of triangle congruence using one of several congruence criteria, such as Side-Angle-Side (SAS), Side-Side-Side (SSS), or Angle-Side-Angle (ASA). We present a generic representation of CPCTC. CPCTC is a powerful tool for establishing relationships and making conclusions about congruent triangles and their corresponding elements, contributing to the precision and rigor of geometric reasoning.īelow is the figure-1.

This principle allows for applying c ongruence relationships to individual parts of triangles and facilitates geometric proofs and deductions. In other words, when two triangles are proven to be congruent through methods such as Side-Angle-Side (SAS), Side-Side-Side (SSS), or Angle-Side-Angle (ASA), the corresponding parts of these congruent triangles can be considered identical in terms of their measures or lengths. Read more Halfplane: Definition, Detailed Examples, and Meaning


 0 kommentar(er)
0 kommentar(er)
